Statistical mechanics
Consider a bath of diatomic molecules and atoms in thermal equilibrium as they repeatedly collide with each other. In a thermally equilibrated microcanonical ensemble all 4 degrees of freedom, electronic, translational, rotational, and vibrational each share 1/4 of the total energy.
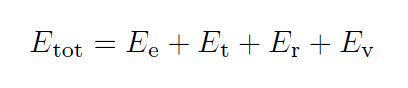
In our work, we only consider two terms, translational and vibrational. The atom-atom collisions are taken to be elastic, therefore, over the duration of the atom-diatom collision, there is no unaccounted change in the Maxwell-Boltzmann speed distribution of the atoms. In our calculations, we consider a canonical ensemble of oscillators initially in thermal equilibrium, exchanging energy with a heat bath. During this energy exchange via inelastic collisions, they temporarily leave thermal equilibrium with the bath.
The equipartition theorem tells us that initially(thermal equilibrium) the average energy for each oscillator is 1/2kT and for each relative translational kinetic energy is 1/2kT . Therefore, just after an inelastic collision, the average for the whole ensemble of both degrees of freedom unequally divides the total kT energy. However, upon relaxation back to thermal equilibrium, equipartition becomes valid again.
A distinction between microcanonical and canonical ensembles is essential here. If we consider the whole system, a microcanonical ensemble, we stay on a constant energy surface in phase space. In microcanonical ensembles, an average overall system temperature may be undefined. We assume no initial thermal equilibrium, as it is necessary that the two degrees of freedom be unequal in energy for any net microscopic dynamics to take place. During this process of relaxation, while thermal equilibrium occurs, an overall system temperature becomes defined.
If we treat the system as a canonical ensemble of oscillators disturbed by collisions, then the opposite occurs. In this case, we begin with a defined temperature in the form of a Boltzmann distribution of initial conditions for the bath of harmonic oscillators. Then the system leaves thermal equilibrium after this statistical ensemble of single inelastic collisions takes place. It is important to note that the whole system is in thermal equilibrium the whole time as the rate at which inelastic collisions are forcing V-T transfers stays equal to the rate at which inelastic collisions are forcing T-V transfers. A formal derivation of a canonical ensemble is done by taking the partial trace of a microcanonical ensemble, integrating out the heat bath degrees of freedom. Since V-T relaxation is usually significantly slower than V-V relaxation, we essentially have a negligable deviation from thermal equilibrium between the two interacting harmonic oscillator baths over the duration of the atom-diatom collision. This is one of many adiabatic approximations needed in this calculation. Among the others are Born-Oppenheimer(electronic adiabaticity), T-T relaxation(assumed to be much slower?) and R-R relaxation(assumed to be much faster).
After an inelastic collision, the original Maxwell-Distribution for the relative speeds will shift in general depending on if the collision is a T-V transfer(a) or a V-T transfer(b).
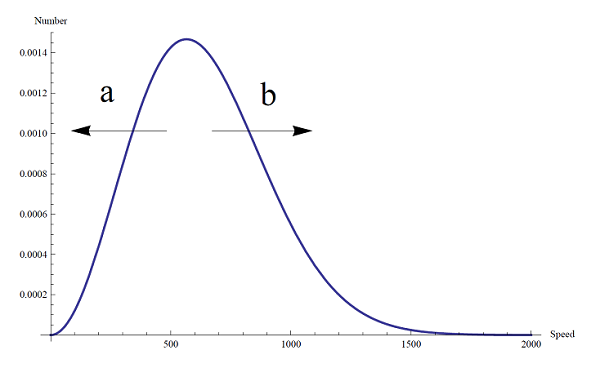
given by the distribution functionn
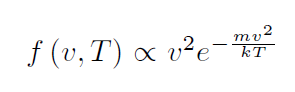
On the other hand, the Maxwell-Boltzmann distribution for the diatomic molecule vibrational states, in general, is shifted the opposite way.
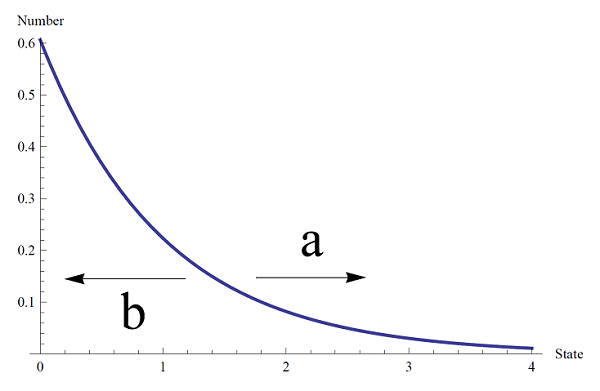
given by the distribution function
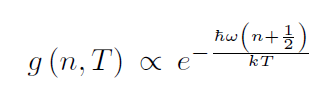
The number of pairs of molecules is the sum of f and of g . Now the goal here is to have these two distributions correlated through time as a “bath” of collisions occur. This might only makes sense using a microcanonical ensemble. In a canonical ensemble, the heat bath provides an infinite amount of energy to draw from. In this sense, a simulaneous time-dependent density of states for these two degrees of freedom is not governed by a limiting energy level.
